24 Wind & Altitude : Effects On Power To Weight Ratio

The following, divided into 3 parts, is a simple physics exploration of wind and altitude's independent effects on power to weight ratio, a subject that has not been treated by any cycling book so far.  Independent effects are important to understand in order to get a feel for how they affect power to weight ratio when acting in combination. The climb chosen is the famous Col du Tourmalet which is featured in this year's Tour. Assumptions in the analysis, for simplicity, include constant speed, constant grade and a formula for frontal area developed by Bassett & Kyle. Validation is done against calculations done by Alex Simmons (see conclusions) for wind vs power to wt results. Power models from Tom Compton's "Analytic Cycling" site and data from prominent researchers are compared with for altitude vs power results.

Courtesy : New Scientist

We have explored VAM calculations  here and here, However, climbing rate by itself does not provide an understanding of the effect of transient wind and altitude on performance. The wind and altitude can make a big difference. On the other hand, one could also argue that the effect of switch-backs and spectators along the road may effectively cancel out the contributions from head and tail winds. I'm not sure how much that contributes but that can be a topic in the comments section.

 
The equation explored in a previous post to understand climbing rate explored only the power needed to increase a rider's potential energy. Lets call this A. If W is total weight of bike and rider in Newtons, Vg ground speed in m/sec :

A = W.V_g.sin[arctan(grade/100)]  Watts

While this is a big piece of the power pie for climbing, there are  couple of other elements to the power equation. One deals with rolling resistance at low speeds. Let's call that B. If Crr is the co-efficient of rolling resistance (typically 0.004 or so for a 100psi tire)

B = W.V_g.Crr.cos[arctan(grade/100)]  Watts

Both A and B are constant if grade and ground velocity are steady.

There's a third element that comes to play which is the effect of wind and density. We can call it C. This is composed of frontal area A and associated drag force. If Cd is the co-efficient of drag (0.9 typical for cyclist on hoods) and this multiplied with area gives drag area, then :

 C = 1/2.C_d.Area

Say very little change happens with the cyclists' drag area (Cd.A where Cd is coefficient of drag and A = area), velocity V and say, density altitude is fixed as p. Let's also assume speed to be steady and gradient of slope to be an average. If  Vw is the wind speed (negative for tailwind) and Vg the ground speed, then not accounting for the tiny drive-train losses in your super efficient bicycle, the power to climb will then be a function of relative velocity as follows :

Power to climb, P  = A + B + C.p.Vg.(Vg + Vw)²  Watts

Let's work with just the third term, calling it Term. Its interesting to see what happens when you normalize Term by the the cyclist's ground speed on the climb. Dividing and multiplying throughout by Vg to change its form but preserve its meaning, we get : 

Term  = C.p.Vg³(1+ Vw/Vg )²

  => K.p.(1+ Vw/Vg )²

where K is defined to be K = C.Vg ³

Vw/Vg is called velocity ratio. Now you can see that when there is no headwind, Vw/Vg =0 and power becomes a function of K and p only. 

PART 1 : Power Variation With Velocity Ratio While Keeping Density Constant

Let's look at how that wind term varies with a change in velocity ratio. The density of the air on Tourmalet is kept uniform for this exercise, at a value of 1.06 kg/m³

When a headwind picks up, the velocity ratio increases from its baseline of  0. The following geometric growth is seen in Term :

• -When headwind is 1/10th of cyclist's forward velocity, Term is an extra 21% compared to no wind condition. 
• -When headwind is 1/4th of cyclist's forward velocity, Term is an extra 56% from no wind condition.  
• -Similarly, when it is half of cyclist's forward velocity, Term is an extra 125% from no wind condition and you must supply 44% more power from case 3).  
• -At an extreme case (assuming you're traveling atleast faster than 5mph), (1+ Vw/Vg) could be highest when the headwind is same as your forward velocity. When that happens, 1+1 = 2 and 2 raised to itself = 4 meaning that all things kept constant, Term is more than 4 times its original value, a 300% increase.  

But this component of power, Term, is added to A and B to produce power, P. Hence, percentage increases shown above don't mean the sum power will also increase by same percentage. So for the last case above, P does not increase by 300%. When one does the math properly (unlike my poorly done first edition of this article), the increase in P from a no-wind condition is actually  :

300% x [ Kp/(A+B+Kp) ]  

Or generally, if all other things are kept the same, 

% increase in power, P%. = [(1+ Vw/Vg )²-1].[Kp/(A+B+Kp)] 

increase in absolute power = P%.(A+B+Kp)

 

We know how to calculate the first part.  The second term, Kp/(A+B+Kp) can be called the Correction Factor. Once this puzzle is solved, we can solve for the proper required increase in power.

Without going into too many details, here are graphs I produced. Maximum height and weight were extracted from the Navy Seals' website. These are some of the fittest people in the world and they were a good choice because it makes no sense to work with data of unhealthy, overweight people. (Note, their dimensions are not like Tour contending emaciated cyclists but it should be close). Frontal area was then calculated using Bassett and colleague's formula that was retweeted by Jonathan Vaughters.  The speed is kept constant at 10mph.  p is kept constant at the average of 1.06 kg/m3. The gradient was uniform at 7.5%, with no change. 

FIG 1 & 2 (above) : First and second plots show the wattage expended to sustain 10mph on the Tourmalet for men and women. The numbers are shown for potential energy and rolling resistance components. Sanity check is to note that wattage needed to counter rolling resistance, for a constant velocity but varying rider weight (shown in red line), is pretty small compared to the wattage needed to fight the grade which is true.

FIG 3 & 4 (above) : Frontal area across riders vary with height and weight. The above plot shows how C varies for men and women according to their dimensions. These dimensions are the maximum accepted in the Navy Seals, who are among the fittest people. Frontal area was calculated using a formula developed by scientist Bessett & Kyle. The area is an approximation ofcourse, and does not account for changing positions on the bike. 

 FIG 5 : The last plot is the power correction factor plot, the puzzle I was seeking to unravel before. Does it make sense? Below, I describe how to use this plot to calculate percentage increase in the total power requirement. 

Going back to the question we were asking earlier : How much increase in power is required to combat a 10 mph headwind (velocity ratio of 1) for a 70 kg male Navy Seal cycling at 10 mph (4.4704 m/sec)? 

Here's how to solve this question. Looking at the figures above, the corresponding numbers for his weight are:

A = 253 W (power due to grade)
B =  15 W (power due to rolling resistance)
C = 0.14 m²
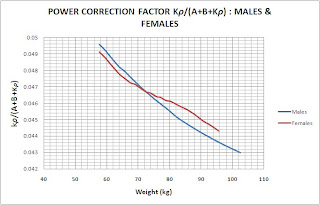
Corresponding correction factor for his weight is ~0.047.
Kp = C.Vg ³p = 0.14(4.4704³).1.06 = 13.25 kg.m²s^-3

Solving using the equations shown earlier in the post,
   

 % increase in power= [(2)²-1].[0.047]~ 0.141 (14.1%)
 increase in absolute power= 0.141(253+15+13.25)~ 40W
 increase in power to weight ratio = 40/70 = 0.57 W/kg

Similarly, we can quantify how much extra boost one would need per kilogram of body weight for different velocity ratios. 

Here's a case scenario for a Tour de France contender on the Tourmalet :
Weight = 65 kg
Height = 1.78m
Power to weight 6 W/kg riding
Desired speed = 10mph.

The plots are shown below : 

 FIG 6 : The operating zone of a Tour contender can, for example, be 6.0 W/kg. This example is for a rider weighing 65 kg. Other parameters assumed are shown in red. In an ideal case, the rider traces a straight horizontal line on the 0 point (y-axis) when there's no wind. Any additional wind will force him to trace horizontal lines as he pushes his body harder. Horizontal trace will move vertically up on the plot with wind change.

The above plot perhaps proves why it pays to prepare the extra mile for July. If 6.4 Watts/kg is what a person needs to win the Tour, perhaps only 6.2 is really needed. The additional 0.2 W/kg is used as insurance, against attacks, accelerations and misfortune winds. Very rarely are riders gifted as such to keep doing this over the course of 3 weeks.

Note : You could also have a tailwind of similar proportions, except that now the (1+ Vw/Vg) term is modified to (1- Vw/Vg). A tailwind of the same proportion as your forward velocity means 1-1 = 0 which multiplied to K effectively cancels your need to combat any wind. It is free speed which you can devote to the terms A and B.  The funny part is that a tailwind never gives you back the "same amount" of speed as the headwind will take away from you. Its a law of nature.

PART 2 : Power Variation With Density Change As Velocity Ratio Remains Constant

Previously, we looked at change in power to weight ratio with change in wind speed. 

Fact of the matter is, air density does change appreciably with altitude as you ascend.  So this term varies with density as climbing progresses :

Term = K.p.(1+ Vw/Vg )²

where K is defined to be K = C.Vg ³ 

The Standard Atmosphere chart, which I pulled out of a book and approximated by a polynomial fit in Excel, tells me the following :

1) At an altitude of 500m (1640 ft), the density is 95% of what it is at sea level. That's a 5% decrease.
2) At an altitude of 1000m (3281 ft), density has decreased by 9.2%. 
3) At an altitude of 1500m (4921 ft), density has decreased by 13.5%. 
4) At an altitude of 2000m (6562ft) , density is now only 82% of its sea level value, a decrease of 18%.

Air density is gently geometrical for the first 1 or 2 miles of the earth's atmosphere. Here is how it behaves :

Like before, I derive the % decrease in power requirements due to altitude with zero headwind (Vw/Vg=0). Let L be the amount representing % of sea level density at any given altitude. If po is sea level density,

 % decrease in power =

[1- (L/100)] / [1+ (A+B)/(Kpo(1+Vw/Vg)² ]

The effect altitude and hence air density has on relieving a rider is very gently geometrical, and not linear. This is proven below. The linear trend-line as you can see doesn't fit 100%.

FIG 7: The number on the y-axis shows how much power to weight ratio one can potentially save while climbing at higher altitude. This is because the higher the altitude, the lesser is the pressure and density of air. This is also why World Hour Record attemps are often done in stadiums that are located high above sea level. Beyond 2500 m ofcourse, advantages in power savings are countered by the thinning of the air. 

FIG 8 :  This diagram simply shows to what degree headwinds and air density contribute to power requirements.

Part 3 : Conclusion & Validation

If wind conditions changed as climbing progresses, density decreases. Whereas wind exponentially increases the power requirements, the density moderately decreases it since you require lesser effort to propel in a less dense medium. But wind is the biggest power soaker.

Here is an interesting chart showing the effects of wind on power to weight ratio given finishing times for Alpe d'Huez. This legendary climb is much more shorter and steeper than Tourmalet but located at lower altitude. It was compiled by Alex Simmons, a cycling coach from Australia.  Perhaps my charts can supplement his or vice versa? It is really well made. 

Simmons' plot can be a sanity check in that power to weight ratio needed with wind on Alpe d'Huez closely correspond with mine for the Tourmalet. I must however add that I have not considered the losses due to drivetrain in my model. For example, a +2.5 mph headwind is a 0.25 velocity ratio. Corresponding additional power to weight ratio (Fig 6) is about 0.13 W/kg from the no wind condition. Assuming a bike is 95% efficient, 0.13/0.95 = 0.14 W/kg. This is my required increase.

Simmons' model for the steeper Alpe d'Huez, on the other hand, says that it should be +0.5 W/kg.  Is this +0.5 W/kg an overestimation, considering that a 2.5 mph wind is classified as just "Light Air" in the Beufort Wind Scale?

If 70 kg rider is producing 6W/kg, he is riding at 420 Watts. A +0.5 W/kg increase means he has to propel himself now at 455 Watts! Phew!

I'm not surprised it is higher than mine since the grade is steeper and my rider weight is 65 kg, although my choice for bike weight is same as his. Also, an important thing to note is that Alpe d'Huez is at lower altitude than the Tourmalet. Hence, it is more than likely that a rider has to put forth more watts on the Alpe than on the Tourmalet. Remember the mildly geometrical reduction effect of density decrease?

Another interesting bit to notice also from the plot above is that a cyclist climbing Alpe d'Huez doesn't get back the same relief from power to weight ratio with a tailwind as the extra amount he has to spend in a headwind. Its funny how that works. Its like climbing hills. You don't get back the time you spent going uphill by going fast downhill.

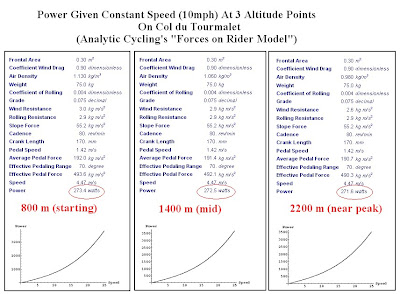
How about verification of the altitude's effect? First, Analytic Cycling's "Forces on Rider" model shows the following power requirements at the bottom, mid point, and top of Tourmalet. Speed was kept constant at 10 mph and all other parameters were made to match my choices before.

You can notice a 1 Watt power demand reduction just by riding from the bottom of the Tourmalet to approximately its midpoint (a 600m elevation change). Not much really in the overall scheme of things since that's a 1W/65kg = 0.003 W/kg decrease. My model (Fig 7) says 0.016 W/kg is the reduction so they're pretty close. From 1400 to 2200m, the reduction is again 1 W, or 0.003 W/kg for the Tom's model. My model says 0.02 W/kg.

Secondly, although data is severely lacking with regards to the effect of altitude on power to weight ratio, a couple of other researchers in the exercise physiology field looked at how aerobic power varied  for 4 groups of runners, not cyclists. They estimated that aerobic power as a percentage of that at sea level, signified by "y", drops off wrt to elevation by the following relationship :

Below, I have plotted those 3 estimations along with mine (65 kg rider producing 6W/kg) upto an altitude of 2200m (the max height of the Tourmalet).

What we see above is apples and oranges. Mine calculates the relief in effort to maintain the same speed due to decrease in altitude density while the others have calculated the decline in power output due to a decline in VO2 max output. They really don't co-relate, do they (advantage vs degradation) ? But is it fair to say that the net effect of both of these phenomena is a curve in-between them?


I must conclude by stating that Alex's theoretical calculations for the wind effect matches closely with mine but this is not the same case for the altitude effect (see above). However, it remains to be said that all our numbers remain to be validated from real powermeter data and CFD simulations for cycling. I'm curious to see how both wind, and high altitude, affect a cyclist in the real world and I'll bet the changes are highly non-linear in nature.  Discussions ongoing at Cycling Forums.

*  *  *